Statistica Sinica 11(2001), 549-552
A NOTE ON CYCLIC ORTHOGONAL DESIGNS
Dennis K. J. Lin and J. Y. Chang
The Pennsylvania State University and Idaho State University
Abstract:
Orthogonality is considered to be one of the most important features
for design of experiment. In this paper, we investigate orthogonal main-effect
designs with cyclic structure in order to ensure the balance among all design
columns. We show that such designs exist for any number of factors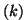 
and
moreover, they are not unique. An explicit form for D-efficiency of cyclic
orthogonal designs is derived. It is shown that D-optimal cyclic design
essentially involves minimizing the tightness of the experimental range. The
minimum tightness D-optimal cyclic orthogonal designs are presented and
tabulated for 
.
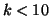Key words and phrases:
D-optimality, Plackett and Burman designs, Regular simplex designs,
T-optimality.